Abstract:
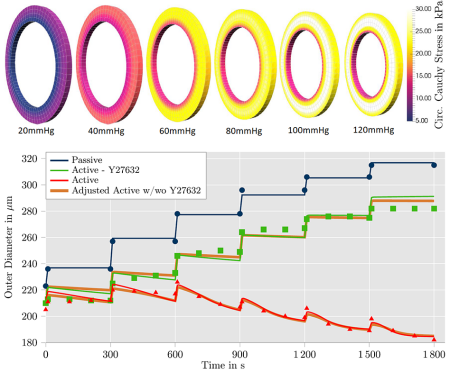
Contrary to striated muscle tissue, smooth muscle cells contract involuntarily to support the functionality of hollow organs such as the bladder or uterus, and protect the tissue from damage by overstretching. In the arterial wall, vascular smooth muscle cells are responsible for the adaptive reaction to changing blood pressure by which the blood vessels actively support the heart in providing sufficient blood supply for varying demands in the supplied tissues. To improve clinical practice in respect to medical and more invasive treatments of cardiovascular diseases, computational simulations of arterial walls in health and disease are considered a promising toolbox. A corresponding mechanical model has to be designed to describe two different stretch-dependent mechanisms which can be observed in smooth muscle cells: a calcium-dependent and a calcium-independent contraction. For the first one, stretch of the SMCs leads to an inlet of calcium ions which activates the myosin light chain kinase (MLCK). The increased activity of MLCK triggers the contractile units of the cells resulting in the contraction on a comparatively short time scale. For the calcium-independent contraction mechanism, stretch-dependent receptors of the cell membrane stimulate an intracellular reaction leading to an inhibition of the antagonist of MLCK, the myosin light chain phosphatase resulting in a contraction on a comparatively long time scale. The capturing of both contraction mechanisms in a chemo-mechanical model allows to describe the experimentally observed contraction of an artery as a reaction to increased internal pressure. This can be considered a crucial aspect of the regulatory mechanism of muscular arteries.
References:
DFG (German Research Foundation) project within the SPP(Priority Program) 2311 "Robust coupling of continuum-biomechanical in silico models to establish active biological system models for later use in clinical applications - Co-design of modeling, numerics and usability"
Cooperation with:
Prof. Dr. Axel Klawonn (University of Cologne)
Prof. Dr. Oliver Rheinbach (TU Bergakademie Freiberg)
Dr. Alexander Heinlein (TU Delft)
Abstract:
This project deals with the modeling of the pharmaco-mechanical interactions that occur in the vasculature. Pharmaceutical agents that directly influence the physical properties of arterial walls are routinely prescribed in the treatment of cardiovascular diseases such as hypertension and atherosclerosis. Understanding the behaviour of healthy and diseased arteries under the influence of drugs can help not only in optimizing treatment but also in the development of new drugs. As a part of this project, suitable models for complex pharmaco-mechanical processes such as drug-induced modifications of smooth muscle activation and remodeling of tissue composition are developed. A robust numerical framework for the solution of the resulting coupled fluid-structure-chemistry problems is developed. Domain decomposition based parallel solvers are used to solve large-scale patient specific problems.
Abstract:
As living biological materials, arterial tissues have the ability to improve their load-bearing behavior by adapting to changes in their mechanobiological environment conditions.
It is for example known that arteries try to compensate for an enduring elevation of the blood pressure by increasing the thickness of their walls.
Besides such an increase of the overall tissue volume, referred to as "growth", a reorganization of the existing tissue components, a so-called "remodeling" process, might occur as a reaction to changes of the loading situation.
Arterial tissues are multi-layered, heterogeneous composites which can be idealized as matrix materials with embedded fibers of varying orientation.
Based on this idealization, a reorientation of the fibers following the local demands can be considered in the context of arterial remodeling.
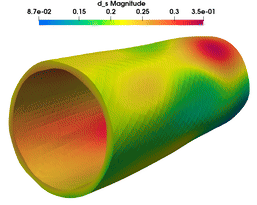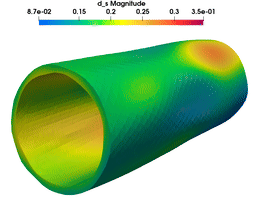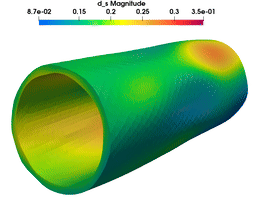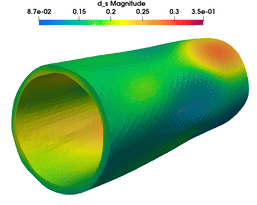
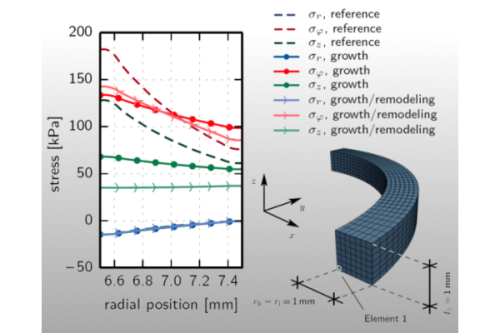
As a consequence of arterial adaptation processes, which tend to reduce strain or stress peaks and/or gradients in the loaded state, residual stresses arise.
For the numerical simulation of arteries, it is necessary to include these commonly unknown, self-balancing stresses, which are present in the unloaded state, since they affect the behavior under external loading.
Modeling arterial adaptation processes allows for an estimation of growth-induced residual stresses.
Moreover, it provides an option to obtain a mechanically motivated estimate for the real distribution of the fiber angles, which is another unknown input quantity in numerical simulations.
References:
DFG (Deutsche Forschungsgemeinschaft) project BA 2823/9-1, in cooperation with SNF (Swiss National Science Foundation) under the D-A-CH agreement
Cooperation with:
A. Klawonn (Universität zu Köln),
A. Quarteroni und S. Deparis (École polytechnique fédérale de Lausanne)
O. Rheinbach (Technische Universität Bergakademie Freiberg),
J. Schröder (Universität Duisburg-Essen)
Abstract:
Transmural stress distributions of in vivo arteries are a major factor driving, e.g., the processes of arteriosclerosis and arteriogenesis. Realistic predictions for transmural stress distributions require a dynamic simulation considering the interaction of the blood flow with the vessel wall. One cannot expect to obtain precise predictions for vessel wall stresses using solid models that do not reflect the global layer structure and the anisotropic fibrous microstructure of the vessel wall. Furthermore, eigenstress distributions in the vessel wall must be taken into account for the analysis of more realistic stress regimes and can be observed to have significant influence on simulations. The fluid structure interaction (FSI) problem is known to be a nontrivial problem especially when nonlinear models are used for the structural part describing the deformation of the arterial wall. In this project, algorithms for the fluid structure interaction are developed based on domain decomposition methods and applied to the computation of realistic transmural stresses in physiological models of arterial walls. The associated systems of coupled nonlinear partial differential equations are to be solved in 3D and on different parallel machines. Moreover, a biologically motivated model for the incorporation of residual stresses is constructed based on nonlocal stress measures.
References:

DFG (Deutsche Forschungsgemeinschaft) project BA 2823/9-1, in cooperation with SNF (Swiss National Science Foundation) under the D-A-CH agreement
Cooperation with:
G. A. Holzapfel (Technische Universität Graz, Österreich)
Abstract:
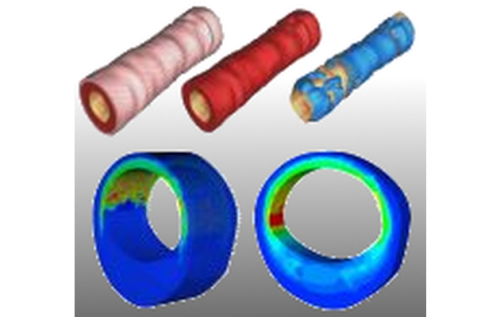
This research project deals with the analysis and the modeling of traumatic degenerations of overstretched arterial walls that occur in therapeutical interventions. The data base for the qualitative and quantitative description of arterial tissues is obtained from biaxial extension tests performed on the tissue components of individual arterial layers loaded far beyond the physiological domain. Such tests enable the analysis of the macroscopic mechanical response of the tissues. In addition, structural analysis techniques such as Fourier transfer infrared spectroscopy and scanning electron microscopy are used to study damage on the smaller length scale. The macroscopic response of the fiber-reinforced tissues is described by a formulation based on micro-mechanical models characterizing the individual tissue components. These models take into account alterations of stochastic distributions of fiber properties as a consequence of the tissue overstretch. In order to obtain a quantitative prediction of the material response the model parameters are adjusted to the performed experiments based on least-square minimization. Finally, the models are validated by comparing finite element calculations with experiments performed on whole arterial wall segments.
References:
In Cooperation with:
L. Perotti (University of Central Florida, USA)
Abstract:
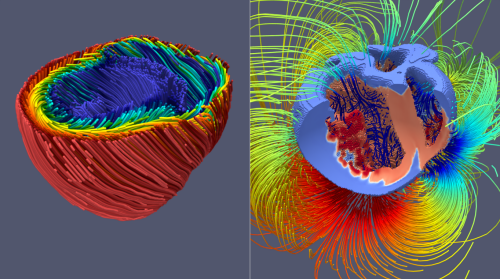
Heart simulations can be a versatile tool in research and precision medicine. For example, we can use virtual tissue models to investigate potential negative and positive effects of drugs on the heart. Another interesting application is the investigation of mechanisms behind heart failure, because computer simulations allow us to observe details which may not be accesible in experiments. Further these models could also be useful in the clinical practice, where heart simulations could for example be used to localize defective tissues, guiding physicians through effective therepeutic interventions and diagnostics.
The goal of this project is to set three corner stones on the road towards practical relevant heart simulations.First, the mathematical models and the implementation of the numerical simulation code must be properly verified and validated against experimental data. This ensures to a certain degree that predictions made by the model are physiologically plausible. Our focus is on electromechanical models of the two large heart chambers, including the cardiac conduction system, which are validated against clinical relevant quantities like ECGs. Once a good model has been constructed, the next important part of the project is to scale up the performance of the simulation, as most of the currently available simulation approaches are known to be very slow. Constructing an efficient method is inherently important in the translation process as not every facility can afford the computational ressources and their associated energy cost.
References:
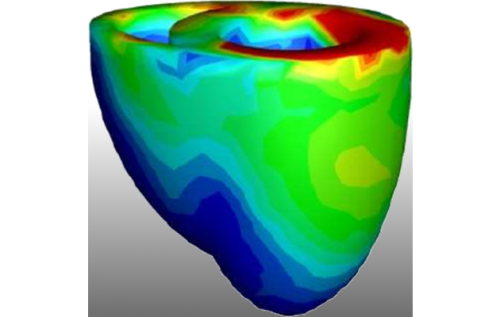
Cooperation with:
L. Perotti (University of California Los Angeles, USA)
B. Klug (University of California Los Angeles, USA)
Abstract:
In order to improve diagnosis techniques to detect diseased tissues, particularly in important organs such as the heart, typically imaging-based methods are often applied. These enable a variety of information including geometry, deformation, distribution of electro-magnetic fields, etc. Based thereon, in this project a highly efficient method is developed to identify distributions of material properties within the heart as a solution of an improved reformulated inverse problem. An important focus is the guaranteed uniqueness of solutions to enable a reliable quantitative analysis.
Cooperations with:
Dr.-Ing. Mike Röllig (Fraunhofer-Institut für Keramische Technologien und Systeme IKTS),
Prof. Dr. rer. nat. habil. E. Spörl (Augenklinik, Universitätsklinikum Carl Gustav Carus an der Technischen Universität Dresden)
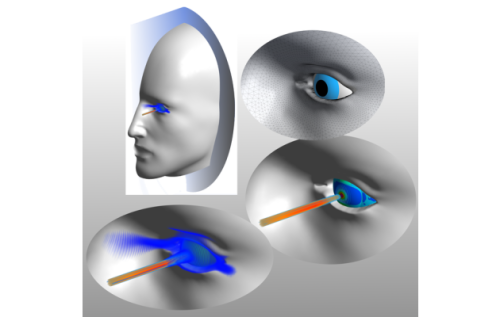
Abstract:
In this research project, methods for the identification of biomechanical parameters of the human eye are to be derived based on numerical calculations. These parameters are of paramount importance in ophthalmology where they reflect pathological processes due to biochemical changes in the tissue structure.
By developing a reliable method for estimating the unknown biomechanical parameters, it is possible to diagnose various eye diseases at an early stage. This is substantial to avoid eye surgery in many cases, as most today's non-surgical treatment procedures only interrupt the progression of the disease and maintain its actual state. Furthermore, because the biomechanics of the eye tissue influences its imaging properties and thus its visual acuity, targeted changes in the biomechanics are usually conducted using techniques such as LASIK to adjust the imaging properties and, consequently, correct the refraction error. Nevertheless, through a successful identification of the patient-specific biomehcanical properties of the eye, more efficient and reliable adjustment and correction procedures can be achieved. Moreover, by a regular estimation of the biomechanical parameters the healing process can be followed and monitored.
This work focuses on the numerical simulation of the system response of the human eye during appropriate medical testing methods. In the first step, different influential biomechanical factors are identified and evaluated through significance analyses. Subsequently, by using these factors, a mathematical model describing the eye behavior is derived. This model will be used in the future in the identification process of the biomechanical properties using inverse analysis methods.
The tasks defined in this project require a realistic definition of external loads as well as a detailed geometric description of the human eye and its boundary conditions. Additionally, they require the derivation of appropriate material models of the individual eye components.
Cooperation with:
J. Guck (Biotechnology Center, Center for Molecular and Cellular Bioengineering, TU Dresden),
C. Werner(Leibniz-Institute for Biofunctional Polymer Materials Dresden)
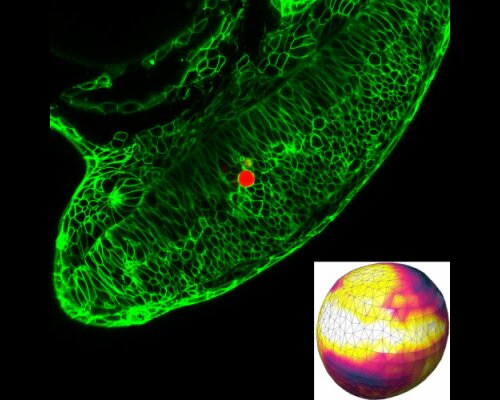
Abstract:
Mechanical stress exerted and experienced by cells during tissue morphogenesis and organ formation plays an important role in embryonic development. While techniques to quantify mechanical stresses in vitro are available, few methods exist for studying stresses in living organisms. In this project, hydrogel microbeads are injected into living tissue and imaged via fluorescence confocal microscopy. A method is developed to reconstruct the stress states of microbeads at different stages of the tissue development. In the long term, a more detailed understanding of the connection of cellular stresses and cell growth can be accomplished, supporting the diagnostics of developmental defects.