Modeling of Aleatoric and Epistemic Uncertainties in the Simulation of Multiphase Steel Structures

DFG (Deutsche Forschungsgemeinschaft) project BA2823/12-1 and BA2823/12-2 within the Priority Program SPP1886  "Polymorphic uncertainty modelling for the numerical design of structures"

Abstract:

In this project we aim to develop methods for the computational analysis of multiphase steel structures taking into account aleatoric and epistemic uncertainties, which are associated with the material's microstructure and mechanical properties. The main goal is to predict the probability of failure (PoF) of an engineering structure in a certified sense by numerically identifying optimal bounds on the PoF, which take into account incomplete or imprecise statistical data. The approach is based on minimizing/maximizing a finite-dimensional parameterization of the PoF in terms of Dirac masses to obtain the sharpest bounds possible. The available statistical data associated with macroscopic material properties enter the optimization problem as constraints. This statistical data will be partially obtained by numerical calculations performed at the microscale and homogenization of the microscopic response. Therein, incomplete data regarding the microstructure is incorporated in terms of bounds on statistical descriptors of higher order that describe the microstructure morphology. For this purpose, statistically similar representative volume elements are considered allowing for an efficient representation of the real microstructure and enabling access to targeted variations of microstructure morphology, which may not necessarily follow explicit probability distributions.

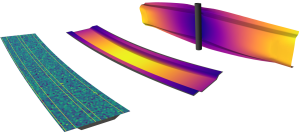
In the second project phase, the extended optimal uncertainty quantification (OUQ) framework developed in the first phase was integrated within a reliability-based design optimization. The optimization target therein is a performance measure of an engineering structure, with can be optimized based on design parameters, whilst a predefined upper threshold on the probability of failure must not be exceeded. Since both the computation of the performance measure as well as the upper bound on the probability of failure are influenced by polymorphic uncertainties, the extended OUQ framework is applied in both cases. Additionally, the extended OUQ framework was combined with fuzzy numbers, which are known from the literature. An important aspect of the project is the incorporation of random fields to model spatially varying material properties, which again introduce another source of uncertainties. The application of the developed optimization method is the maximization of the dissipated energy of a car front bumper in a crash scenario by optimizing the positions of laser-hardened traces within the front bumper.

• N. Miska and D. Balzani [2019], "Quantification of uncertain macroscopic material properties resulting from variations of microstructure morphology based on statistically similar volume elements: application to dual-phase steel microstructures", Computational Mechanics, Vol. 64, pp. 1621-1637.
• K. Kremer, P. Edler, N. Miska, F. Leichsenring, D. Balzani, S. Freitag, W. Graf, M. Kaliske and G. Meschke [2019], "Modeling of structures with polymorphic uncertainties at different length scales", Surveys for Applied Mathematics and Mechanics (GAMM-Mitteilungen), Published online.
• N. Miska and D. Balzani [2022], "Method for the Analysis of Epistemic and Aleatory Uncertainties for a Reliable Evaluation of Failure of Engineering Structures", International Journal for Uncertainty Quantification, Vol. 12(6), pp. 23-45.
• N. Miska and D. Balzani [2023], "Reliability-Based Design Optimization of Laser-Hardened Traces in a Car Front Bumper Under the Influence of Polymorphic Uncertainties", Proceedings in Applied Mathematics and Mechanics, Published online.
• N Miska, S. Freitag and D. Balzani [2024], "Nested Optimal Uncertainty Quantification for an Efficient Incorporation of Random Fields - Application to Sheet Metal Forming", International Journal for Uncertainty Quantification, Vol. 14(1), pp. 89-106.

Robust and Efficient Finite Element Discretizations for Higher Order Gradient Formulations

DFG (Deutsche Forschungsgemeinschaft) project within the SPP (German Priority Program) 1748    "Reliable Simulation Techniques in Solid Mechanics. Development of Non-standard Discretization Methods, Mechanical and Mathematical Analysis"

Cooperation with:
Mira Schedensack (Universität Leipzig)

Abstact:
In solid mechanics, the development of advanced numerical models that can capture phenomena such as length-scale dependent constitutive behavior or material softening due to micro-damage are a field of ongoing research. More and more, nonlocal approaches including higher-order derivatives are considered, because not only are they able to model these phenomena but also to regularize geometrical singularities that lead to spurious mesh sensitivities when using standard local models. However, the finite element implementation of these nonlocal gradient models is a challenging task. So far, state of the art approaches are either incompatible with classical approximation schemes because of higher continuity requirements or relatively cost intensive due to a high number of independent variables. Within this project, a new approach is investigated: With the help of the rotation-free condition of gradient fields, the displacement gradient is taken as the only independent variable. This way, on the one hand the high continuity requirement is relaxed enabling incorporation in existing FE-software packages and on the other hand enhanced efficiency towards existing mixed formulations is expected.

• J.Riesselmann, J.Ketteler, M.Schedensack and D.Balzani [2019], "Three-field mixed finite element formulations for gradient elasticity at finite strains", GAMM-Mitteilungen, Published online, DOI: 10.1002/gamm.202000002 
• J.Riesselmann, J.Ketteler, M.Schedensack and D.Balzani [2019], "A New C0-Continuous FE-Formulation for Finite Gradient Elasticity", PAMM Proc. Appl. Math. Mech., Vol. 19, No. 1
• J.Riesselmann, J.Ketteler, M.Schedensack and D.Balzani [2019], "C0 continuous finite elements for gradient elasticity at finite strains", Proc. of 8th GACM Colloq. on Comp. Mech., pp. 27-30
• J.Riesselmann, J.Ketteler, M.Schedensack and D.Balzani [2021], "Rot-free mixed finite elements for gradient elasticity at finite strains", International J Numer Methods in Eng.,
• J.Riesselmann, J.Ketteler, M.Schedensack and D.Balzani [2021], "Rot-free finite elements for gradient-enhanced formulations at finite strains", Proceedings in Applied Mathematics and Mechanics (PAMM), 20:1,
• J. Riesselmann and D. Balzani. [2022] “Finite Element Formulations For Gradient Damage at Finite Strains”. In: Current Trends and Open Problems in Computational Mechanics. Ed. by F. Aldakheel. et al., Springer, pp. 443–452.
• J. Riesselmann, J.W. Ketteler, M. Schedensack, and D. Balzani [2022] “Robust and Efficient Finite Element Discretizations for Higher-Order Gradient Formulations”. In: Non-standard Discretisation Methods in Solid Mechanics. Springer, 2022, pp. 69–90
• P. Junker, J. Riesselmann and D.Balzani [2022], “Efficient and robust numerical treatment of a gradient-enhanced damage model at large deformations”, International J Numer. Methods in Eng.
• J. Riesselmann and D. Balzani [2023] “A simple and efficient lagrange multiplier based mixed finite element for gradient damage”. In: Computers and Structures 281

Calculation of Optimal Bounds for the Probability of Failure in Soft Biological Tissues

Cooperation with:
M. Ortiz (California Institute of Technology CALTECH, Pasadena, USA)

Abstract:
The computational simulation of soft biological tissues is of high interest to medical doctors since these calculations can provide additional information leading to an improvement of diagnosis and treatment. In particular for surgical intervention as e.g. during balloon-angioplasty the quantification of probabilities of failure is important. However, due to a general lack of experimental data, classical uncertainty quantification methods cannot be applied because the full probability distribution of the random input data (as e.g. stiffness of the material) can not be assumed to be given. Then the calculation of bounds on the probability of failure may be a suitable approach which at least incorporates the information that may be assumed to be known, as e.g. the mean of the probability distribution. In this project we focus on the calculation of optimal bounds which are obtained by solving minimization/maximization problems in the probability space.

Novel finite element technologies for anisotropic media

DFG (Deutsche Forschungsgemeinschaft) project within the SPP (German Priority Program) 1748   "Reliable Simulation Techniques in Solid Mechanics. Development of Non-standard Discretization Methods, Mechanical and Mathematical Analysis"

Cooperation with:
J. Schröder (Universität Duisburg-Essen),
P. Wriggers (Leibniz Universität Hannover)

Abstract:
Considering solid mechanics, a majority of the problems can be solved using the standard Galerkin method. Although this method is used as a standard tool for predicting the behavior of a variety of engineering structures, certain problems limit the applicability. In general, incompressible and/or anisotropic materials could lead to not well-posed formulations. Finite-element formulations, which are available today using a purely volumetric-isochoric split, are not sufficient for anisotropic materials. Therefore, in this research project, the primary goal is to develop new finite-element formulations as a suitable basis for the stable calculation of complex materials in nonlinear applications. In order to achieve this goal new ideas have to be pursued since there is no obvious approach available at the moment to overcome these difficulties. Therefore, we follow three main strategies:

• Different approximations of the kinematic quantities entering the isotropic and anisotropic parts of the free energy function provide the possibility to relax the constraints arising from anisotropy. In this approach the structure of the polyconvex energy function is preserved.
• Special approximations of the minors of the deformation gradient lead to mixed formulations suitable for more general polyconvex strain energy functions. Thereby the ansatz spaces for the mixed variables approximating the minors are balanced.
• The VFEM, which was formulated so far only for small strain problems, is extended to large strains based on isotropic/anisotropic polyconvex strain energy functions. Thereby, the advantage to discretize non-convex regions using VFEM is exploited for the application to highly distorted deformed meshes.

References

• J. Schröder, N. Viebahn, D. Balzani and P. Wriggers [2016], "A novel mixed finite element for finite anisotropic elasticity; the ska-element - simplified kinematics for anisotropy", Computer Methods in Applied Mechanics and Engineering, Vol. 310, pp. 475-494.
• J. Schröder, P. Wriggers and D. Balzani [2011], "A new Mixed Finite Element based on Different Approximations of the Minors of Deformation Tensors", Computer Methods in Applied Mechanics and Engineering, Vol. 200, pp. 3583–3600.

Robust Numerical Approximation Schemes for Tensor-Valued Derivatives of Higher Order

Cooperation with:
M. Tanaka (Toyota R&D Laboratories, Inc., Yokomichi, Japan)

Abstract:
For the numerical simulation of nonlinear problems in the context of finite elements the stresses and their derivatives, the tangent moduli, are required for the calculation of element residual vectors and stiffness matrices. For complex materials these are typically time-consuming to be derived and implemented. For certain numerical methods as e.g. the FE²-scheme an analytical tangent does not even exist, since the stresses are numerically calculated. One approach to reduce development time here is to use numerical approximations of these derivatives. The main disadvantage of the classical approach where forward differences are considered is that they suffer from approximation errors and round-off errors. Contrary to this, the methods developed here are based on complex-step derivative approximations and hyper dual numbers. Thereby, robust approximation schemes are developed that lead to computer accuracy although being insensitive with respect to perturbation values. The resulting approximation schemes are successfully applied to hyperelastic, inelastic and thermo-plastic problems at finite strains.

• M. Tanaka, M. Fujikawa, D. Balzani, J. Schröder [2014], "Robust numerical calculation of tangent moduli at finite strains based on complex-step derivative approximation and its application to localization analysis, Computer Methods in Applied Mechanics and Engineering, Volume 269, Pages 454-470,
• M. Tanaka, T. Sasagawa, R. Omote, M. Fujikawa, D. Balzani, J. Schröder [2015], "A highly accurate 1st- and 2nd-order differentiation scheme for hyperelastic material models based on hyper-dual numbers", Computer Methods in Applied Mechanics and Engineering, Volume 283, Pages 22-45.